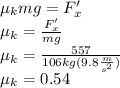Answer:
a)
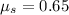
b)
Step-by-step explanation:
Static friction is when the body is at rest or is about to move while kinetic friction is when the body is already in motion. According to Newton's second law:
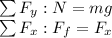
a) In this case, the static friction must be equal to the horizontal force to set the clock in motion:

b) In this case, the kinetic friction is equal to the horizontal force that keep the clock moving with constant velocity: