Question

A 75.0-kg cross-country skier is climbing a 3.0° slope at a constant speed of 2.00 m/s and encounters air resistance of 25.0 N.

a) Find his power output for work done against the gravitational force and air resistance.

b) What average force does he exert backward on the snow to accomplish this?

c) If he continues to exert this force and to experience the same air resistance when he reaches a level area, how long will it take him to reach a velocity of 10.0 m/s?

Answer 1

a) 12.01 W

b) 63.506 N

c) 11.82 s

Step-by-step explanation:

Given data:

mass = 75 kg

slope of inclination = 3 degree

speed of skier is 2.00 m/s

air Resistance = 25 N

a) From the given information we have following relation

`F_n = mgsin\theta + F_a`

`F_n = 75* 9.81 sin 3 + 25 = 63.506 N`

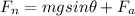
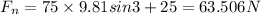
Power output

`P =F_n * v`

`P = 63.506 * 2 = 127.01 W`

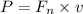
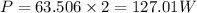
b) average force can be calculate as

`F_n = mgsin\theta + F_a`

`F_n = 75 * 9.81 sin 3 + 25`

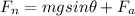
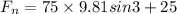
F_n = 63.506 s

c)

force of acceleration is f = ma

solving for acceleration

`a = (F)/(m)`

`a = (63.506)/(75)`

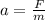
a = 0.846 m/s^2

we know acceleration is given as
`a =(v -u)/(t)`

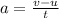
solving for time t

`t = (v-u)/(a)`

`t =(10-0)/(0.846)`

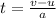
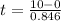
t = 11.82 s