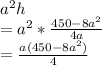Question

I built a storage shed in the shape of a rectangular box on a square base. The material that I used for the base cost $4 per square foot, the material for the roof cost $2 per square foot, and the material for the sides cost $2.50 per square foot; and I spent $450 altogether on material for the shed. Express the volume of the shed as a function of the (length of each) side of the square base

Answer 1

`(a(450-8a^2))/(4)`

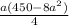
is the volume in terms of side a

Explanation:

Given that you I built a storage shed in the shape of a rectangular box on a square base. The material that I used for the base cost $4 per square foot, the material for the roof cost $2 per square foot, and the material for the sides cost $2.50 per square foot; and I spent $450 altogether on material for the shed.

Let a be the side of square and h be the height

Total cost of materials = cost for floor + cost for roof + cost for sides

= area of floor (4) + lateral area (2.50)+roof area (2)

`=4a^2+4ah+4a^2\\=8a^2+4ah = 450`
`h = (450-8a^2)/(4a)`

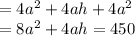
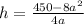
Now coming to volume

Volume = V = lbh =
`a^2 h\\= a^2*(450-8a^2)/(4a) \\=(a(450-8a^2))/(4)`