Question

Law School According to the Law School Admission Council, in the fall of 2007, 66% of law school applicants wereaccepted to some law schooL4 The training program LSATisfaction claims that 163 of the 240 students trained in 2006were admitted to law school. You can safely consider these trainees to be representative of the population of law schoolapplicants. Has LSAfisfaction demonstrated a real improvement over the national average?a) What are the hypotheses?b) Check the conditions and find the P-value.c) Would you recommend this program based on what you see here? Explain.

Answer 1

a)
`H_(0): p = 0.66\\H_A: p > 0.66`

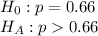
b) P-value = 0.2650

c) No, this programme will not be recommended as there is no real improvement over the national average.

Explanation:

We are given the following in the question:

Sample size, n = 240

p = 66% = 0.66

Alpha, α = 0.05

Number of students admitted to law school , x = 163

a) First, we design the null and the alternate hypothesis

`H_(0): p = 0.66\\H_A: p > 0.66`

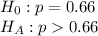
This is a one-tailed(right) test.

Formula:

`\hat{p} = (x)/(n) = (163)/(240) = 0.6792`

`z = \frac{\hat{p}-p}{\sqrt{(p(1-p))/(n)}}`

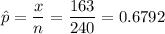
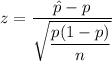
Putting the values, we get,

`z = \displaystyle\frac{0.6792-0.66}{\sqrt{(0.66(1-0.66))/(240)}} = 0.6279`

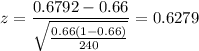
b) Now, we calculate the p-value from the table.

P-value = 0.2650

c) Since the p-value is greater than the significance level, we fail to reject the null hypothesis and accept the null hypothesis.

Thus, there is no real improvement over the national average.

No, this programme will not be recommended as there is no real improvement over the national average.