Question

Ultra-thin semiconductor materials are of interest for future nanometer-scale transistors, but can present undesirably high resistance to current flow. How low must the resistivity of a semiconductor material be, to ensure that the resistance of a 2nm-thick, 10nm-long, 100nm-wide region does not exceed 100 ohms?

Answer 1

p = 2*10^(-7) ohm m

Step-by-step explanation:

The resistivity and Resistance relationship is:

`p = (R*A)/(L)`

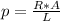
For lowest resistivity with R < 100 ohms.

We need to consider the possibility of current flowing across minimum Area and maximum Length.

So,

Amin = 2nm x 10 nm = 2 * 10^(-16) m^2

Lmax = 100nm

Using above relationship compute resistivity p:

`p = (100*2*10^(-16))/(100*10^(-9)) \\\\p = 2 * 10^(-7)`

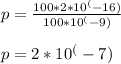
Answer: p = 2*10^(-7) ohm m