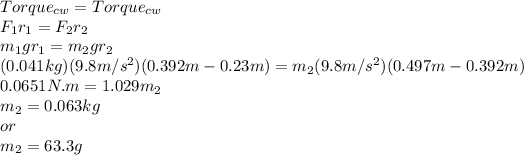Question

A meter stick is found to balance at the 49.7-cm mark when placed on a fulcrum. When a 41.0-gram mass is attached at the 23.0-cm mark, the fulcrum must be moved to the 39.2-cm mark for balance. What is the mass of the meter stick?

Answer 1

mass of the meter stick=0.063 kg

or

mass of the meter stick=63.3 g

Step-by-step explanation:

Given data

m₁=41.0g=0.041kg

r₁=(39.2 - 23)cm

r₂=(49.7 - 39.2)cm

g=9.8 m/s²

To find

m₂(mass of the meter stick)

Solution

The clockwise and counter-clockwise torques must be equal if the meter stick is in rotational equilibrium

`Torque_(cw)=Torque_(cw)\\F_(1)r_(1)=F_(2)r_(2)\\ m_(1)gr_(1)=m_(2)gr_(2)\\(0.041kg)(9.8m/s^(2) )(0.392m-0.23m)=m_(2)(9.8m/s^(2))(0.497m-0.392m)\\0.0651N.m=1.029m_(2)\\m_(2)=0.063 kg\\or\\m_(2)=63.3g`