Question

Coulomb's Law: Two electrons are 20.0 mm apart at closest approach. What is the magnitude of the maximum electric force that they exert on each other? (e = 1.60 × 10-19 C, k = 1/4πε0 = 9.0 109 N ∙ m2/C2)

Answer 1

The maximum electric force is
`5.76*10^(-25)\ N`

Step-by-step explanation:

Given that,

Distance = 20.0 mm

We need to calculate the maximum electric force that they exert on each other

Using formula of electric force

`F=(1)/(4\pi\epsilon_(0))(q^2)/(r^2)`

Where, q = charge

r = radius

Put the value into the formula

`F=9*10^(9)*((1.6*10^(-19))^2)/((20.0*10^(-3))^2)`

`F=5.76*10^(-25)\ N`

Hence, The maximum electric force is
`5.76*10^(-25)\ N`

Answer 2

F = 5.76 x 10⁻²⁵ N

Step-by-step explanation:

given,

distance between electron,r = 20 mm = 0.02 m

charge of electron, q = 1.6 x 10⁻¹⁹ C

k = 9 x 10⁹ N.m²/C²

Electric force magnitude

using electric force formula = ?

`F = (kq^2)/(r^2)`

`F = (9* 10^9* (1.6* 10^(-19))^2)/((0.02)^2)`

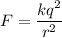
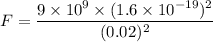
F = 5.76 x 10⁻²⁵ N

hence, the electric force exerted by the electron on reach other is equal to F = 5.76 x 10⁻²⁵ N.