Question

In triangle RST, RT = 4, ST = 8, and TX bisects < RTS.

Which of the following proportions must be true?
RX/ST = TRIXS
TX/XR = ST/RT
RX/RT = SX/ST
RX/RS = XS/ST

Answer 1

B is the correct answer!

TX/XR=ST/RT

Explanation:

Answer 2

`(TX)/(XR)=(ST)/(RT)`

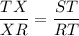
Explanation:

In triangle RST, TX bisects angle RTS.

Angle bisector theorem says that an angle bisector of a triangle will divide the opposite side into two segments that are proportional to the other two sides of the triangle.

Hence, TX divides side RS in the ratio:

`(TX)/(XR)=(ST)/(RT)`

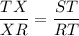
Therefore, option B is true.