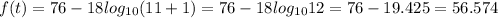Answer:
a)
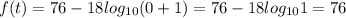
b)
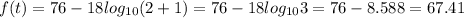
c)
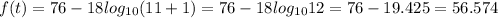
Explanation:
For this case we know that the average scores for the class are given by the following model:
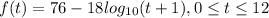
Where t is in months. The graph attached illustrate the function for this case
And for this case we can answer the questions like this:
Part a
We just need to replace t =0 into the model and we got:
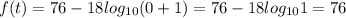
Part b
We just need to replace t =2 into the model and we got:
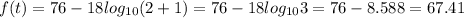
Part c
We just need to replace t =11 into the model and we got: