Question

Asteroid 1 moving 8km/s right asteroid 2 moving 16 km/s left before the crash. After the crash asteroid 1 is moving 4 km/s left and changed speed bu 12km/s. Asteroid 2 moving 2km/s left and changed speed by 14km/s

Two asteroids crashed. The crashed caused both asteroids to change speed. Scientist want to use the change in speed and motion to figure out which asteroid has more mass. Based on the information in the diagram. which statement is correct? In your answer explain what the forces were like and why the asteroids changed motions

Asteroid 1 has more mass than asteroid 2
Asteroid 1 and asteroid 2 are the same mass
Or asteroid 1 has lees mass than asteroid 2

Answer 1

Answer: Asteroid 1 has more mass than asteroid 2

Step-by-step explanation:

Answer 2

Answer: Asteroid 1 has more mass than asteroid 2

Step-by-step explanation:

Let's begin by explaining this crash between both asteroids is known as an elasic collision, since both followed opposite paths after they crashed.

In addition, according to the conservation of momentum law it is stated the following:

"If two objects or bodies are in a closed system and both collide, the total momentum of these two objects before the collision will be the same as the total momentum of these same two objects after the collision".

This means the momentum before the collision (
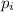`p_(i)`) is equal to the momentum after the collision (
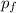`p_(f)`). Hence the momentum is conserved:

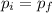`p_(i)=p_(f)` (1)

Before the crash:

`p_(i)=m_(1)V_(1)+m_(2)V_(2)` (2)

Where:

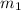`m_(1)` is the mass of the first asteroid

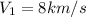`V_(1)=8 km/s` is the velocity of the first asteroid

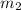`m_(2)` is the mass of the second asteroid

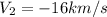`V_(2)=-16 km/s` is the velocity of the second asteroid

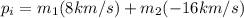`p_(i)=m_(1)(8 km/s)+m_(2)(-16 km/s)` (3)

After the crash:

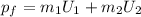`p_(f)=m_(1)U_(1)+m_(2)U_(2)` (4)

Where:

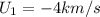`U_(1)=-4 km/s` is the final velocity of the first asteroid

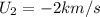`U_(2)=-2 km/s` is the final velocity of the second asteroid

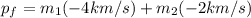`p_(f)=m_(1)(-4 km/s)+m_(2)(-2 km/s)` (5)

Substituting (3) and (5) in (1):

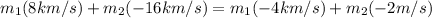`m_(1)(8 km/s)+m_(2)(-16 km/s)=m_(1)(-4 km/s)+m_(2)(-2 m/s)` (6)

Grouping similar terms:

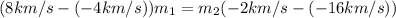`(8 km/s-(-4 km/s))m_(1)=m_(2)(-2 km/s-(-16 km/s))` (7)

Then:

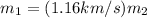`m_(1)=(1.16 km/s) m_(2)` (8) This means
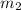`m_(2)` must be multiplied by 1.16 km/s in order to make this side of the equation equal to
`m_(1)`.

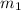Hence:

`m_(1)>m_(2)`

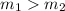
Asteroid 1 has more mass than asteroid 2