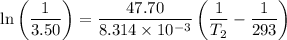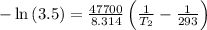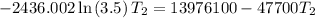Question

A certain substance has a heat of vaporization of 47.70 kJ/mol. At what kelvin temperature will the vapor pressure be 3.50 times higher than it was at 293k?=______K

Answer 1

`T_2 = 313\ K`

Step-by-step explanation:

he expression for Clausius-Clapeyron Equation is shown below as:

`\ln P = \frac{-\Delta{H_(vap)}}{RT} + c`

Where,

P is the vapor pressure

ΔHvap is the Enthalpy of Vaporization

R is the gas constant (8.314×10⁻³ kJ /mol K)

c is the constant.

For two situations and phases, the equation becomes:

`\ln \left( (P_1)/(P_2) \right) = (\Delta H_(vap))/(R) \left( (1)/(T_2)- (1)/(T_1) \right)`

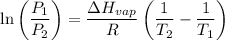
Given:

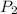`P_2` = 3.50
`P_1`

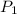
`T_1` = 293 K

`\Delta \:H_(vap)=47.70\ kJ/mol`

So,

`\ln \left( (P_1)/(3.50* P_1) \right) = (47.70)/(8.314* 10^(-3)) \left( (1)/(T_2)- (1)/(293) \right)`

`\ln \left( (1)/(3.50) \right) = (47.70)/(8.314* 10^(-3)) \left( (1)/(T_2)- (1)/(293) \right)`

`-\ln \left(3.5\right)=(47700)/(8.314)\left((1)/(T_2)-(1)/(293)\right)`

`-2436.002\ln \left(3.5\right)T_2=13976100-47700T_2`

`T_2 = 313\ K`