Question

Of all the luggage handled by the airlines at JFK in the last 10 years, 5% was lost or stolen or damaged. For a randomly selected sample of 400 pieces of outgoing luggage at JFK International Airporta) Would it be unusual for 10 pieces of luggage to be lost?b) The mean and standard deviation of the binomial distribution is given byc) Would 40 pieces of luggage lost be an unusual occurrence?d) Find the probability that less than 6 pieces of luggage are lost or stolen.

Answer 1

a) 10 pieces of luggage to be lost is not likely because its z-score is -2.29

b) mean=20 and standard deviation=4.359

c) 40 pieces of luggage lost is very extreme given that in average 5% of the luggage is lost.

d) The probability that less than 6 pieces of luggage are lost or stolen is 0.0007

Explanation:

Let p be the proportion of stolen or damaged luggages of all the luggage handled by the airlines at JFK in the last 10 years.

p=0.05 (5%)

b) Mean and standard deviation of the binomial distribution is given as:

• Mean= n×p

• Standard Deviation =
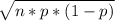  `√(n*p*(1-p))` where n is the sample size

Thus,

Mean=400×0.05=20 and

Standard Deviation=
`√(400*0.05*0.95)` ≈ 4.359

a) We should calculate z-score of 10 pieces of luggage lost to decide if it is unusual.

Z-score can be calculated as follows:

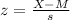`z= (X-M)/(s)}` where

• X = 10 pieces of luggage lost

• M is the mean lost luggage (20 pieces)
• s is the standard deviation (4.359)

Thus,
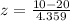`z= (10-20)/(4.359)` ≈ -2.29

This is unusual because it is in the 1st percentile and 99% of the possible number of lost luggage are higher than this score.

c) 40 pieces of lost luggage is also unusual because its z score is:

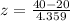`z= (40-20)/(4.359)` ≈ 4.59, in the 100th percentile, that is very extreme.

d) the probability that less than 6 pieces of luggage are lost or stolen

= P(z<z*) where z* is the z-score of 6 pieces of luggage are lost or stolen

That is
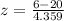`z= (6-20)/(4.359)` ≈ -3.21

And P(z<3.21)= 0.0007