Answer:
The period of oscillation is 1.33 sec.
Step-by-step explanation:
Given that,
Mass = 275.0 g
Suppose value of spring constant is 6.2 N/m.
We need to calculate the angular frequency
Using formula of angular frequency
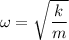
Where, m = mass
k = spring constant
Put the value into the formula

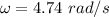
We need to calculate the period of oscillation,
Using formula of time period
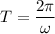
Put the value into the formula
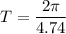
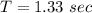
Hence, The period of oscillation is 1.33 sec.