Question

Based on the thermodynamic properties provided for water, determine the amount of energy released for 155.0 g of water to go from 39.0 °C to -36.5°C. Property Melting point Boiling point AHfus AHvap Cp (s) Value 0.0 100.0 6.01 40.67 37.1 75.3 33.6 Units oC kJ/mol kJ/mol J/mol.oc J/mol C mol oC Cp (g)

Answer 1

Answer : The amount of energy released will be, -88.39 kJ

Solution :

The process involved in this problem are :

`(1):H_2O(l)(39.0^oC)\rightarrow H_2O(l)(0^oC)\\\\(2):H_2O(l)(0^oC)\rightarrow H_2O(s)(0^oC)\\\\(3):H_2O(s)(0^oC)\rightarrow H_2O(s)(-36.5^oC)`

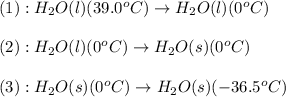
The expression used will be:

`\Delta H=[m* c_(p,l)* (T_(final)-T_(initial))]+m* (-\Delta H_(fusion))+[m* c_(p,l)* (T_(final)-T_(initial))]`

where,

`\Delta H` = heat available for the reaction = ?

m = mass of water = 155.0 g

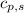`c_(p,s)` = specific heat of solid water =
`2.01J/g^oC`

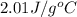
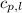`c_(p,l)` = specific heat of liquid water =
`4.18J/g^oC`

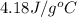
= enthalpy change for fusion =
`6.01kJ/mole=6010J/mole=(6010J/mole)/(18g/mole)J/g=333.89J/g`

Molar mass of water = 18 g/mole

Now put all the given values in the above expression, we get:

`\Delta H=[155.0g* 4.18J/g^oC* (0-(39.0))^oC]+155.0g* -333.89J/g+[155.0g* 2.01J/g^oC* (-36.5-0)^oC]`

`\Delta H=-88392.625J=-88.39kJ`

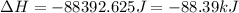
Therefore, the amount of energy released will be, -88.39 kJ