Question

Emil's backpack weighs six and three eights pounds. He removes a book that weighs three fourth pound. Then he removes a book that weighs one half pound .How much does Emil's back pack weigh now

Answer 1

Emil's back pack weigh now
`5(1)/(8)\ pounds`.

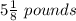Explanation:

Given:

Total Weight of backpack =
`6(3)/(8)\ pounds`

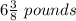
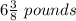`6(3)/(8)\ pounds` can be Rewritten as
`(51)/(8)\ pounds`

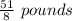
Weight of backpack =
`(51)/(8)\ pounds`

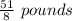
Weight of Book 1 =
`(3)/(4)\ pound`

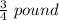
Weight of Book 2 =
`(1)/(2)\ pound`

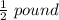
We need to find weight of back pack after removing books.

Solution:

Now we can say that;

weight of back pack after removing books can be calculated by Subtracting Weight of Book 1 and Weight of Book 2 from Total Weight of backpack.

framing in equation form we get;

weight of back pack after removing books =
`(51)/(8)-(3)/(4)-(1)/(2)`

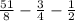
Now to solve the equation we will first make the denominator common using LCM.

weight of back pack after removing books =
`(51*1)/(8*1)-(3*2)/(4*2)-(1*4)/(2*4)=(51)/(8)-(6)/(8)-(4)/(8)`

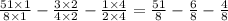
Now the denominators are common so we will solve the numerator.

weight of back pack after removing books =
`(51-6-4)/(8)=(41)/(8)\ pounds \ \ OR \ \ 5(1)/(8)\ pounds`

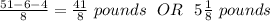
Hence Emil's back pack weigh now
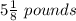`5(1)/(8)\ pounds`.