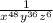Question

Simplifying an Expression with the Numerator and the Denominator Raised to a Power

Answer 1

First, Second, and fourth answers :)

Explanation:

Answer 2

The answer to your question is the second option
`(1)/(x^(48)y^(36)z^(6))`

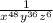
Explanation:

Expression

`[((x^(2)y^(3))^(-2))/((x^(6)y^(3)z)^(2))]^(3)`

Process

1.- Divide the fraction in numerator and denominator

a) Numerator

[(x²y³)⁻²]³ = (x⁻⁴y⁻⁶)³ = x⁻¹²y⁻¹⁸

b) Denominator

[(x⁶y³z)²]²= (x¹²y⁶z²)³ = x³⁶y¹⁸z⁶

2.- Simplify like terms

a) x⁻¹²x⁻³⁶ = x⁻⁴⁸

b) y⁻¹⁸y⁻¹⁸= y⁻³⁶

c) z⁻⁶

3.- Write the fraction

`(1)/(x^(48)y^(36)z^(6))`