Question

Find the area of triangle

Answer 1

So we see one large triangle, that can be split into two triangles split by the thin dotted line:

• the First triangle --> has a hypotenuse of 26 and a leg of 11
• the Second triangle --> has a hypotenuse of 42

Let us find the other leg of the First triangle which also happens to be the height of the larger triangle, using the Pythagorean theorem

`a^2+b^2=c^2\\a^2 + 11^2 = 26^2\\a^2 = 26^2-11^2=555\\a=23.56`

Thus the height of the entire triangle is 23.56 which also happens to be one of the legs of the Second triangle

`a^2+b^2=c^2\\a^2+(23.56)^2=42^2\\a^2 = 1764-555=1209\\a = 34.77`

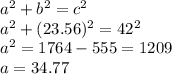
Having found the other leg of the Second Triangle, we can find the base of the larger triangle is:

--> 34.77 + 11 = 45.77

The height as found in the first initial step is 23.56 thus the area is

Area =
`(1)/(2)*b*h=(1)/(2)*23.56*45.77=539.17`

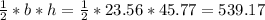
Hope that helps!