To solve this problem we will apply the linear motion kinematic equations. In which the speed is defined as the distance traveled in a certain period of time. In turn we must emphasize that 1 light year is equivalent to
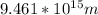 . Mathematically the speed is described as,
. Mathematically the speed is described as,
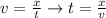
Replacing,
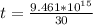
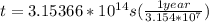
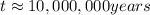
Therefore the correct answer is D.