Question

Two identical cylindrical vessels with their bases at the same level each contain a liquid of density 1.23 g/cm3. The area of each base is 3.89 cm2, but in one vessel the liquid height is 0.993 m and in the other it is 1.76 m. Find the work done by the gravitational force in equalizing the levels when the two vessels are connected.

Answer 1

Step-by-step explanation:

Work done by gravity is given by the formula,

W =
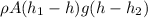`\rho A (h_(1) - h)g (h - h_(2))` ......... (1)

It is known that when levels are same then height of the liquid is as follows.

h =
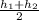`(h_(1) + h_(2))/(2)` ......... (2)

Putting value of equation (2) in equation (1) the overall formula will be as follows.

W =
`(1)/(4) \rho gA(h_(1) - h_(2))^(2))`

=
`(1)/(4) * 1.23 g/cm^(3) * 9.80 m/s^(2) * 3.89 * 10^(-4) m^(2)(1.76 m - 0.993 m)^(2))`

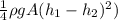
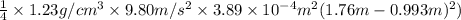
= 0.689 J

Thus, we can conclude that the work done by the gravitational force in equalizing the levels when the two vessels are connected is 0.689 J.