Question

A wheel with a 0.10-m radius is rotating at 35 rev/s. It then slows uniformly to 15 rev/s over a 3.0-s interval.

a. What is the angular acceleration of a point on the wheel?
b. how to do and explain will give lifesaver!

Answer 1

The angular acceleration of a point on the wheel is
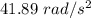`41.89\ rad/s^2` and it is decelerating.

Step-by-step explanation:

It is given that,

Radius of the wheel, r = 0.1 m

Initial angular velocity of the wheel,
`\omega_i=35\ rev/s=219.91\ rad/s`

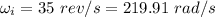
Final angular velocity of the wheel,
`\omega_f=15\ rev/s=94.24\ rad/s`

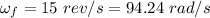
Time, t = 3 s

We need to find the angular acceleration of a point on the wheel. It is given by the rate of change of angular velocity divided by time taken. It is given by :

`\alpha =(\omega_f-\omega_i)/(t)`

`\alpha =((94.24-219.91)\ rad/s)/(3\ s)`

`\alpha =-41.89\ rad/s^2`

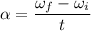
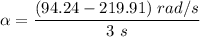
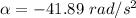
So, the angular acceleration of a point on the wheel is
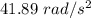`41.89\ rad/s^2` and it is decelerating. Hence, this is the required solution.