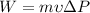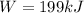Question

6 A simple ideal Rankine cycle with water as the working fluid operates between the pressure limits of 4 MPa in the boiler and 20 kPa in the condenser and a turbine inlet temperature of 700°C. The boiler is sized to provide a steam flow of 50 kg/s. Determine the power produced by the turbine and consumed by the pump.

Answer 1

To solve this problem we will start by differentiating the values in each of the states of matter. Subsequently through the thermodynamic tables we will look for the values related to the entropy, enthalpy and respective specific volumes. Through the relationship of Power defined as the product between mass and enthalpy and mass, specific volume and pressure, we will find the energetic values in the two states investigated. We will start defining the states

State 1

`T_1 = 700\°C`

`P_1 = 4 Mpa`

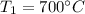
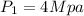
From steam table

`h_1 =3906.41 KJ/Kg`

`s_1 = 7.62 KJ/Kg.K`

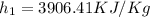
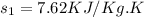
Now

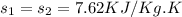`s_1 = s_2 = 7.62 KJ/Kg.K` As 1-2 is isentropic

State 2

`P_2 = 20 Kpa`

`s_2 = 7.62 KJ/Kg \cdot K`

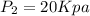
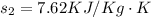
From steam table

`h_2 = 2513.33 KJ/Kg`

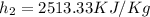
PART A) The power produced by turbine is the product between the mass and the enthalpy difference, then

`Power = m * (h_1-h_2)`

`P = (50)(3906.41 - 2513.33)`

`P = 69654kW`

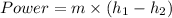
b) Pump Work

State 3

`P_3 = 20 Kpa`

`\upsilon= 0.001 m^3/kg`

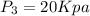
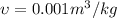
The Work done by the pump is

`W= m\upsilon \Delta P`

`W = (50)(0.001)(4000-20)`

`W = 199kJ`