Question

Happy Paws charges $20.00 plus $3.50 per hour to keep a dog during the day. Woof Watchers charge

$11.00 plus $4.75 per hour. Complete the equation and solve it to find for how many hours the totale
of the services is equal. Use the variable h to represent the number of hours.
The equation is
hours.
The total cost of the services will be equal at​

Answer 1

The system of equation is
`\left \{ {{20+3.5h} \atop {11+4.75h}} \right.`.

The total cost of the services will be equal at 7.2 hrs.

Explanation:

Let the number of hours be represented by 'h'.

Given:

Happy Paws charges :

Fixed charge = $20.00

Per hour charge = $3.50.

Now we know that;

Total charges is equal to Fixed charge plus Per hour charge multiplied by number of hours.

framing in equation form we get;

Total charges =
`20+3.5h \ \ \ \ equation \ 1`

Also Given:

Woof Watchers charge

Fixed charge = $11.00

Per hour charge = $4.75.

Now we know that;

Total charges is equal to Fixed charge plus Per hour charge multiplied by number of hours.

framing in equation form we get;

Total charges =
`11+4.75h \ \ \ \ equation \ 2`

Hence the system of equation is
`\left \{ {{20+3.5h} \atop {11+4.75h}} \right.`.

We need to find the number of hours when both charges are equal.

`20+3.5h=11+4.75h`

Combining the like terms we get;

`4.75h-3.5h=20-11\\\\1.25h = 9`

Dividing both side by 1.5 we get;

`(1.25h)/(1.25)=(9)/(1.25)\\\\h = 7.2`

Hence The total cost of the services will be equal at 7.2 hrs.

Total charges of Happy Paws =
`20+3.5h =20 +3.5*7.2 = \$45.2`

Total charges of Woof Watchers =
`11+4.75h = 11+4.75*7.2 = \$45.2`

Answer 2

A) The number of hours for service charge of both to be equal is 7 hours 12 minutes

B) Total cost of services is $45.2

Explanation:

Given as :

The fixed charge taken by Happy Paws = $20

The moving charge taken by Happy Paws = $3.50 per hours

The fixed charge taken by Woof Watchers = $11

The moving charge taken by Woof Watchers = $4.75 per hours

Let The number of hours for service charge of both to be equal = n hours

Let The total cost of services = $A

According to question

For service charge of both to be equal

The fixed charge taken by Happy Paws + moving charge taken by Happy Paws × number of hours for service charge = The fixed charge taken by Woof Watchers + moving charge taken by Woof Watchers × number of hours for service charge

i.e $20 + $3.50 × n = $11 + $4.75 × n

Or, $20 - $11 = $4.75 × n - $3.50 × n

Or, $9 = $1.25 × n

∴ n =
`(9)/(1.25)`

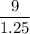
i.e n = 7.2 hours

So, The number of hours for service charge of both to be equal = n = 7 hours 12 minutes

Hence , The number of hours for service charge of both to be equal is 7 hours 12 minutes . Answer

Again

Total cost of services = $11 + $4.75 × n

i.e A = $11 + $4.75 × 7.2

Or, A = $11 + $34.2

Or. A = $45.2

So, Total cost of services = A= $45.2

Hence, Total cost of services is $45.2 Answer