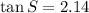Answer:
Therefore,
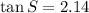
Explanation:
Given:
ΔSRQ is a Right angle triangle at ∠R = 90°
SR = 36 ....Adjacent side of ∠S
RQ = 77 ...Opposite side of ∠S
To Find:
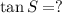
Solution:
ΔSRQ is a Right angle triangle at ∠R = 90° ..Given
By Tangent Identity we have
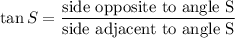
Substituting the values we get
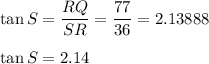 ...Rounded to the nearest hundredth
...Rounded to the nearest hundredth
Therefore,