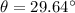Question

A 866 kg car is traveling at 16.7 m/s around a banked curve with a radius

of 50 m. At what angle would the car have to be banked so that no
friction is required?

Answer 1

Answer:
`29.64 \°`

Step-by-step explanation:

The expression to calculate the angle
`\theta` of an ideally banked curve in which friciton is not needed is:

`\theta=tan^(-1)((V^(2))/(r.g))`

Where:

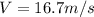`V=16.7 m/s` is the car's velocity

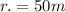`r.=50 m` is the radius of the curve

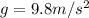`g=9.8 m/s^(2)` is the acceleration due gravity

As you may see, this angle does not depend on the mass of the car.

Solving with the given values:

`\theta=tan^(-1)(((16.7 m/s)^(2))/((50 m)(9.8 m/s^(2))))`

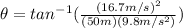
Finally:

`\theta=29.64 \°`