Answer: 0.57
Step-by-step explanation:
Let's begin by explaining that two angles are complementary when they sum is
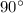 :
:
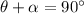
Having this clear, let's find
 from the following trigonometric function:
from the following trigonometric function:
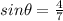
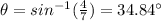
Now that we have the value of
 , let's find
, let's find
 :
:

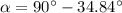
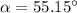 This is the complement of angle
This is the complement of angle

Then, the cosine of the complement
 is:
is:
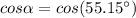
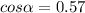 This is the answer
This is the answer