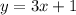Answer:
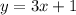
Explanation:
From the question, we are given;
- The equation y = 3x + 2
- A point (-1, -2)
We are required to determine the equation of a line parallel to the given line and passing through the point (-1, -2)
- We are going to determine the gradient of the line first; when an equation is written in the form of y = mx + c, where m is the gradient.
y = 3x + 2, m₁ = 3
- But, m₁ = m₂ for parallel lines
- Therefore, the gradient of the line in question, m₂ is 3
- With the gradient, m₂=3 , and a point (-1, -2) we can get its equation;
Taking another point (x, y)...
Then;
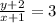
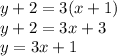
Therefore; the equation of the line is