Question

A store is having a sale on jelly beans and almonds. For 6 pounds of jelly beans and 2

pounds of almonds, the total cost is $16 . For 3 pounds of jelly beans and 5 pounds of almonds, the total cost is $13. Find the cost for each pound of jelly beans and each pound of almonds.

Answer 1

The cost of each pound of jelly beans is $ 2.25 and cost of each pound of almond is $ 1.25

Solution:

Let the cost of each pound of jelly beans is "a"

Let the cost of each pound of almond is "b"

For 6 pounds of jelly beans and 2 pounds of almonds, the total cost is $16

Therefore, we frame a equation as:

6 pounds of jelly beans x cost of each pound of jelly beans + 2 pounds of almonds x cost of each pound of almond = 16

`6 * a + 2 * b = 16`

6a + 2b = 16 --------- eqn 1

For 3 pounds of jelly beans and 5 pounds of almonds, the total cost is $13

Therefore, we frame a equation as:

3 pounds of jelly beans x cost of each pound of jelly beans + 5 pounds of almonds x cost of each pound of almond = 13

`3 * a + 5 * b = 13`

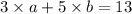
3a + 5b = 13 ----------- eqn 2

Let us solve eqn 1 and eqn 2

Multiply eqn 2 with 2

6a + 10b = 26 --------- eqn 3

Subtract eqn 1 from eqn 3

6a + 10b = 26

6a + 2b = 16

( - ) -------------------------

8b = 10

`b = (10)/(8) = 1.25`

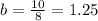
b = 1.25

Substitute b = 1.25 in eqn 1

6a + 2(1.25) = 16

6a + 2.5 = 16

6a = 13.5

a = 2.25

Thus cost of each pound of jelly beans is $ 2.25 and cost of each pound of almond is $ 1.25