Answer:
9.2 cm.
Explanation:
A pyramid has a horizontal square base of 12 cm. The vertex of the pyramid is 7 cm vertically above the base.
So, the height of the pyramid is 7 cm and half-length i.e. half base is equal to
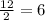 cm.
cm.
Now, the slant height of a pyramid is the altitude of the side triangles.
Therefore, the length of the slant height will be equal to
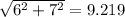 cm ≈ 9.2 cm. (Answer)
cm ≈ 9.2 cm. (Answer)