Answer:
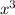 - 2
- 2
 - 11x + 12 = 0
- 11x + 12 = 0
Explanation:
Since the zeros of the polynomials are -3 , 4 and 1 , then the equation will be in the form:
(x+3)(x-4)(x-1) = 0
Expanding (x+3)(x-4) , we have
 - 4x + 3x - 12
- 4x + 3x - 12
⇒
 -x - 12
-x - 12
combining with (x-1) , we have
(
 -x - 12) ( x -1 ) = 0
-x - 12) ( x -1 ) = 0
expanding , we have :
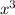 -
-
 -
-
 + x - 12x + 12 = 0
+ x - 12x + 12 = 0
⇒
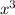 - 2
- 2
 - 11x + 12 = 0
- 11x + 12 = 0