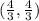Question

The top of a ladder is 4m up a vertical wall.The bottom is 2m from the wall.The coordinate axes are the wall and the horizontal ground.

a)Find the equation representing the ladder.
b)A square box just fits under the ladder.Find the coordinates of the point where the box touches the ladder.

Answer 1

Part a)
`y=-2x+4`

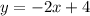
Part b) The coordinates of the point are
`((4)/(3),(4)/(3))`

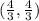
Explanation:

Part a) Find the equation representing the ladder

we have the ordered pairs

(0,4) and (2,0)

Find the slope

`m=(0-4)/(2-0)=-2`

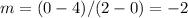
Find the equation of the line in slope intercept form

`y=mx+b`

we have

`m=-2\\b=4`

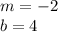
substitute

`y=-2x+4`

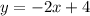
Part b) A square box just fits under the ladder.Find the coordinates of the point where the box touches the ladder.

If the box is a square

the x-coordinate of the point where the box touches the ladder must be equal to the y-coordinate

x=y

`y=-2x+4`

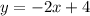
substitute

`x=-2x+4\\3x=4\\x=(4)/(3)`

`y=x=(4)/(3)`

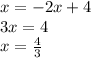
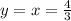
therefore

The coordinates of the point are
`((4)/(3),(4)/(3))`