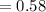Question

A raft is made of 14 logs lashed together. Each log is 42 cm in diameter and a length of 6.4 m. 42% of the log volume is above the water when no one is on the raft. Determine the following: the specific gravity of the logs.

Answer 1

Step-by-step explanation:

Given

No of logs
`n=14`

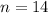
diameter of log
`d=42\ cm`

Length of log
`L=6.4\ m`

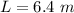
42 % of log volume(V) is above water when no one is on raft

so 58 % of log volume(V) is submerged in the water

Weight of 14 log

`W=14* \rho _(log)* V* g`

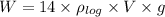
Buoyancy force on 14 logs
`F_b=14* \rho _(water)* 0.58V* g`

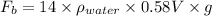
as system is in equilibrium so

`W=F_b`

`14* \rho _(log)* V* g=14* \rho _(water)* 0.58V* g`

`\rho _(log)=0.58\rho _(water)`

`(\rho _(log))/(\rho _(water))=0.58`

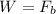
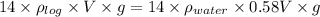
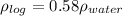
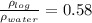
Specific gravity of log
`=0.58`