Question

Two cards are drawn from a standard deck without replacement. What is the probability that the first card is a club and the second card is red? (

Answer 1

Probability to draw a club and second card is red without replacement will be
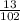`(13)/(102)` or 12.75%

Explanation:

A standard deck of cards contains number of cards = 52

And a standard deck contains number of club = 13

Now the probability of first card to be a club will be

`P_(1)=\frac{\text{Number of club in a deck}}{\text{Total number of cards}}`

=
`(13)/(52)=(1)/(4)`

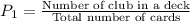
Now this card is separated from the deck.

Therefore, number of remaining cards = 52 - 1 = 51

Second card has been drawn from the deck without replacement.

Since number of red cards in the deck = 13 + 13 = 26

Probability of the second card drawn =
`\frac{\text{Number of red cards}}{\text{Number of remaining cards}}`

`P_(2)=(26)/(51)`

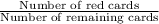
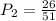
Now probability that the first card is a club and the second card is red =
`P_(1)* P_(2)=(1)/(4)* (26)/(51)`

=
`(13)/(102)`

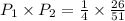
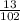
Or
`(13)/(102)* 100=12.75%`%

Therefore, probability to draw a club and second card is red without replacement will be
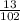`(13)/(102)` or 12.75%