Answer:
r = 1.45 Å
Step-by-step explanation:
given,
λ = 1.436 Å
θ = 20.62°
d = a
n = 2
metal gold crystallizes in a face centered cubic unit cell
Radius of the gold atom = ?
using Bragg's Law
n λ = 2 d sin θ
2 x 1.436 Å = 2 a sin 20.62°
a = 4.077 Å
We know relation of radius for face centered cubic unit cell
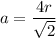
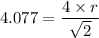
r = 1.45 Å
the radius of a(n) gold atom. is equal to 1.45 Å