Answer:
The values of x that makes these vectors orthogonal are x = 2 and x = 4.
Step-by-step explanation:
Orthogonal vectors
Suppose we have two vectors:
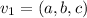

Their dot product is:
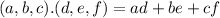
They are ortogonal is their dot product is 0.
Solving quadratic equations:
To solve this problem, we are going to need tosolve a quadratic equation.
Given a second order polynomial expressed by the following equation:
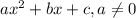 .
.
This polynomial has roots
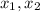 such that
such that
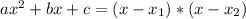 , given by the following formulas:
, given by the following formulas:

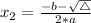
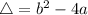
Find all values of x such that (4, x, −6) and (2, x, x) are orthogonal.
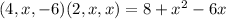
These vectors are going to be orthogonal if:
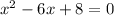
This is a quadratic equation, in which
 . So
. So
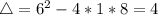

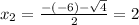
The values of x that makes these vectors orthogonal are x = 2 and x = 4.