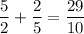Question

A number added to its reciprocal is 2 9/10. Find the number and show your work.

Answer 1

`\displaystyle x=(5)/(2), x=(2)/(5)`

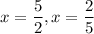
Explanation:

Quadratic Equations

The quadratic equation has the following general form

`ax^2+bx+c=0`

It can be solved by several methods, including the well-known quadratic formula

`\displaystyle x=(-b\pm √(b^2-4ac))/(2a)`

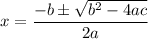
The question requires us to find a number that added with its reciprocal results 2 9/10. If x is the required number

`\displaystyle x+(1)/(x)=2\ (9)/(10)=(29)/(10)`

Operating

`\displaystyle (x^2+1)/(x)=(29)/(10)`

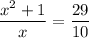
Rearranging

`10(x^2+1)=29x`

`10x^2-29x+10=0`

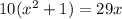
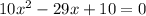
Comparing with the general quadratic equation, we have

`a=10,\ b=-29,\ c=10`

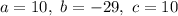
Using the formula

`\displaystyle x=(29\pm √((-29)^2-4(10)(10)))/(2(10))`

`\displaystyle x=(29\pm √(441))/(20)=(29\pm 21)/(20)`

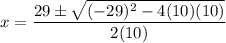
It gives us two possible solutions

`\displaystyle \boxed{x=(5)/(2), x=(2)/(5)}`

Both are valid solutions since

`\displaystyle (5)/(2)+(2)/(5)=(29)/(10)`