Question

Water flowing through both a small pipe and a large pipe can fill a water tank in 4 hours. Water flowing through the small pipe alone can fill the tank in 15 more hours. How many hours would it take to fill the tank using only the small pipe?

Answer 1

Answer with explanation:

As is the question the answer would be 19 hours, and the key to solving it is in the phrase in 15 more hours, basically what they are saying is that the small pipe takes 15 hours more than both the big and the small to fill the tank. Since both pipes working together can fill the tank in 4 hours we need to add 4 and 15 to solve the problem.

If the question is how many hours would it take to fill the tank using only the big pipe? Then we could solve t for the following equation:

`(1)/(4+15) + (1)/(t) = (1)/(4)`

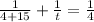
Getting as a result: 5.06

Note that the equation is the result of taking the rate of the small pipe (what we solved before), plus the unknown rate of the big one equals the rate of both.