Question

The practical limit to an electric field in air is about 3.00 × 10^6 N/C . Above this strength, sparking takes place because air begins to ionize.

(a) At this electric field strength, how far would a proton travel before hitting the speed of light (100% speed of light) (ignore relativistic effects)?

(b) Is it practical to leave air in particle accelerators?

Answer 1

(a) x=157 m

(b) No

Step-by-step explanation:

Given Data

Mass of proton m=1.67×10⁻²⁷kg

Charge of proton e=1.6×10⁻¹⁹C

Electric field E=3.00×10⁶ N/C

Speed of light c=3×10⁸ m/s

For part (a) distance would proton travel

Apply the third equation of motion

`(v_(f))^(2) =(v_(i))^(2)+2ax`

In this case vi=0 m/s and vf=c

so

`c^(2)=(0)^(2)+2ax\\ c^(2)=2ax\\x=(c^(2) )/(2a)`

`x=(c^(2))/(2a)--------Equation (i)`

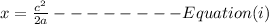
From the electric force on proton

`F=qE\\where\\ F=ma\\so\\ma=qE\\a=(qE)/(m)\\`

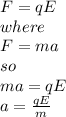
put this a(acceleration) in Equation (i)

So

`x=(c^(2) )/(2(qE/m))\\ x=(mc^(2))/(2qE) \\x=((1.67*10^(-27))*(3*10^(8))^(2) )/(2*(1.6*10^(-19))*(3*10^(6)))\\ x=157m`

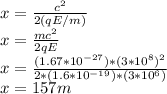
For part (b)

No the proton would collide with air molecule