Answer:
Number of bacteria after 100 days is 1237.
Explanation:
Since bacterial growth is a geometrical sequence.
Therefore, their population after time t will be represented by the expression
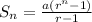
Where a = first term of the sequence
r = common ratio of the sequence
n = duration or time
Since first term of the sequence = number of bacteria in the start = 1
Common ratio = r = (1 + 0.04) = 1.04
![S_(100)=(1[(1.04)^(100)-1)])/(1.04-1)](https://img.qammunity.org/2021/formulas/mathematics/high-school/hq5dw2i69jtn5kk6rbbar3obnms2lakiqv.png)
=
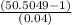
= 1237.64 ≈ 1237 [Since bacteria can't be in fractions]
Therefore, number of bacteria after 100 days is 1237.