Question

3) x2+ y2 - x + 3y - 42 = 0
X+y=4

Answer 1

The solution is
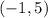`(-1,5)` and
`(7,-3)`

Explanation:

The expression is
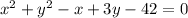`x^(2) +y^(2) -x+3y-42=0` and
`x+y=4`

Using substitution method we can solve the expression.

Let us substitute
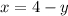`x=4-y` in
`x^(2) +y^(2) -x+3y-42=0`

`(4-y)^(2) +y^(2) -(4-y)+3y-42=0`

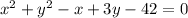
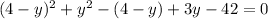
Expanding and simplifying the expression, we get,

`\begin{array}{r}{16-8 y+y^(2)+y^(2)-4+y+3 y-42=0} \\{2 y^(2)-4 y-30=0}\end{array}`

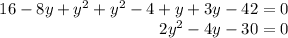
Let us use the quadratic equation formula to solve this equation,

`\begin{aligned}y &=(4 \pm √(16-4(2)(-30)))/(2(2)) \\&=(4 \pm √(16+240))/(4) \\&=(4 \pm 16)/(4) \\y &=1 \pm 4\end{aligned}`

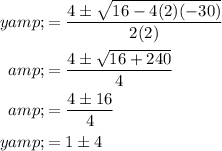
Thus,
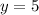`y=5` and
`y=-3`

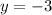
Substituting y-values in the equation
`x+y=4`, we get the value of x.

For
`y=5` ⇒
`x=4-5=-1`

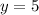For
`y=-3` ⇒
`x=4+3=7`

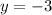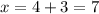
Thus, the solution set is
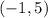`(-1,5)` and
`(7,-3)`