Question

The null hypothesis is that the true proportion of the population is equal to .40. A sample of 120 observations revealed the sample proportion "p" was equal to .30. At the .05 significance level test to see if the true proportion is in fact different from .40.

(a) What will be the value of the critical value on the left?
(b) What is the value of your test statistic?
(c) Did you reject the null hypothesis?
(d) Is there evidence that the true proportion is different from .40?

Answer 1

There is enough evidence to support the claim that the true proportion is in fact different from 0.40

Explanation:

We are given the following in the question:

Sample size, n = 120

p = 0.4

Alpha, α = 0.05

First, we design the null and the alternate hypothesis

`H_(0): p = 0.4\\H_A: p \\eq 0.4`

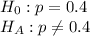
This is a two-tailed test.

Formula:

`\hat{p} = 0.3`

`z = \frac{\hat{p}-p}{\sqrt{(p(1-p))/(n)}}`

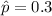
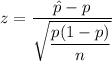
Putting the values, we get,

`z = \displaystyle\frac{0.3-0.4}{\sqrt{(0.4(1-0.4))/(120)}} = -2.236`

Now,
`z_(critical) \text{ at 0.05 level of significance } = \pm 1.96`

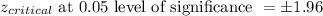
Since,

The calculated z-statistic does not lies in the acceptance region, we fail to accept the null hypothesis and reject it. We accept the alternate hypothesis.

Thus, there is enough evidence to support the claim that the true proportion is in fact different from 0.40