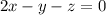Question

Find an equation for the plane tangent to the surface given by z = x 2 + y 4 + e xy at the point (1, 0, 2).

Answer 1

Explanation:

Let

`f(x,y,z) = z-x^2-y^4-e^(xy)`.

The partial derivatives of this function are

`(\partial f)/(\partial x) (x,y,z) = ye^(xy) - 2x \\ (\partial f)/(\partial y) (x,y,z) = xe^(xy) - 4y^3 \\(\partial f)/(\partial z) (x,y,z) = 1`

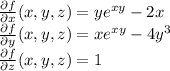
The tangent plane equation through a point
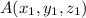`A(x_1,y_1,z_1)` is given by
`f'_x (x_1,y_1,z_1)(x-x_1) + f'_y(x_1,y_1,z_1)(y-y_1) + f'_z(x_1,y_1,z_1)(z-z_1) = 0`

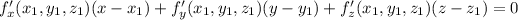
In this case, we have

`x_1 = 1, y_1 = 0, z_1 = 2.`

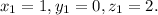
The values of the partial derivatives in this point are

`(\partial f)/(\partial x) (1,0,2) = 0 \cdot e^(0) - 2\cdot 1 = -2 \\ (\partial f)/(\partial y) (1,0,2) = 1 \cdot e^(0) - 0 = 1 \\ (\partial f)/(\partial y) (1,0,2) = 1`

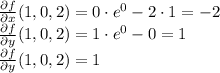
So, the equation is

`-2(x-1) + 1 \cdot (y-0) + 1\cdot (z-2) = 0`

Therefore, the equation for the plane tangent to the surface at the point
`(1,0,2)` is given by

`2x-y-z = 0`