Question

Two plastic spheres each with radius 1.30 cm and mass 9.75 g, have uniform charge densities of 0.100 C/m^3 and -0.100 C/m^3, respectively. The spheres are released from rest when their centers are 80.0 cm apart. What is the speed of each sphere at the moment they collide? Ignore polarization of the spheres.

Answer 1

To develop this problem we will proceed to use the principle of energy conservation. For this purpose we will have that the change in the electric potential energy and kinetic energy at the beginning must be equal at the end. Our values are given as shown below:

`m = 9.75g = 0.00975kg`

`r = 1.3cm = 0.013m`

`q_1 = 0.1 C/m^3 * (4)/(3) \pi r^3`

`q_1 = 9.2*10^(-7)C`

`q_2 = -9.2*10^(-7)C`

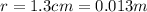
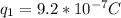
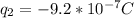
Applying energy conservation equations

`U_1+K_1 = U_2+K_2`

`(k q_1q_2)/(d) +0 = (kq_1q_2)/(2r)+ (1)/(2) (2m)v^2`

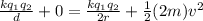
Replacing,

`9*10^(9) (9.2*10^(-7))^2((1)/(0.026)-(1)/(0.8)) = v^2`

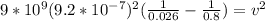
Solving for v,

`v = 9.2*10^(-7) ((9*10^9)/(0.00975)((1)/(0.026)-(1)/(0.8)))^(1/2)`

`v = 5.4 m/s`

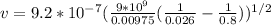
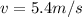
Therefore the speed of each sphere at the moment they collide is 5.4m/s