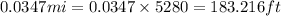Question

A van traveling at a speed of 36.0 mi/h needs a minimum of 50.0 ft to stop. If the same van is traveling 69.0 mi/h, determine its minimum stopping distance (in ft), assuming the same rate of acceleration.

Answer 1

Van will stop after a distance of 183.216 ft

Step-by-step explanation:

We have given initial speed of the van = 36 mi/hr

As the van finally stops so final velocity v = 0 mi/hr

Distance after which van stop = 50 ft

As 1 ft = 0.000189 mi

So 50 ft
`=50* 0.000189=0.00945mi`

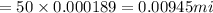
From third equation of motion
`v^2=u^2+2as`

`0^2=36^2+2* a* 0.00945`

`a=-68571.42mi/hr^2`

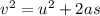
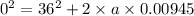
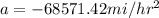
In second case u = 69 mi/hr

And acceleration is same

So
`0^2=69^2-2* 68571.42* s`

`s=0.03471mi`

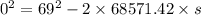
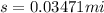
As 1 mi = 5280 ft

So
`0.0347mi=0.0347* 5280=183.216ft`