Question

A firm believes a product’s sales volume (S) depends on its unit selling price (P) as S = $100 – P. The production cost C is $917 + 9S. Determine the sales volume (S) at which the firm’s profit is a maximum. (Hint: calculate derivative of the profit function with respect to the price)

Answer 1

$45.5

Step-by-step explanation:

For a firm, profit is equal to total revenue minus total cost. This can be represented mathematically as follows:

π = R - C ................................................................................................... (1)

Where π represents profit, R represents total revenue and C represents total cost.

Total revenue (R) is unit selling price (P) multiply by the sales volume (S). This is represented mathematically as follows:

R = P * S ...................................................................................................... (2)

From the question, sales volume is given as follows:

S = $100 – P .............................................................................................. (3)

We then substitute for S in equation (2) and solve as follows:

R = P($100 – P) = $100P –
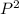`P^(2)` ............................................................... (2a)

From the question, C is given as:

C = $917 + 9S .............................................................................................. (4)

We then substitute for S in equation (4) and solve as follows:

C = $917 + 9($100 – P) = $917 + $900 – 9P = $17 – 9P .................. (4a)

To derive the profit function, the solution for R in equation (2a) and the solution for C in equation (4a) are substituted into equations (1) above and then solved as follows:

π = ($100P –
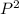`P^(2)` ) – ($17 – 9P) = $100P –
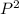`P^(2)` – $17 + 9P

= $100P + 9P –
`P^(2)` – $17

π = $109P –
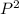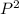`P^(2)` – $17 ................................................................................ (1a)

Equation (1a) is the profit function. The calculation of the derivative of the profit function (1a) with respect to the price is obtained as follows:

dπ/dP = $109 – 2P = 0 ................................................................................. (5)

From equation (5), we can solve for P as follows:

$109 – 2P = 0

$109 = 2P

2P = $109

P = $109/2

P = $54.5 ............................................................................................................ (6)

To determine the sales volume, we substitute 54.5 in equation (6) for P in the product’s sales volume (S) in equation (3) and we then solve as follows:

S = $100 – $54.5 = $45.5

Therefore, the sales volume (S) at which the firm’s profit is a maximum is $45.5.