Answers:
a)
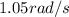
b)
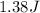
Step-by-step explanation:
a) Final angular velocity :
Before solving this part, we have to stay clear that the angular momentum
 is conserved, since we are dealing with circular motion, then:
is conserved, since we are dealing with circular motion, then:
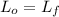
Hence:
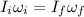 (1)
(1)
Where:
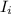 is the initial moment of inertia of the system
is the initial moment of inertia of the system
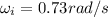 is the initial angular velocity
is the initial angular velocity
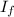 is the final moment of inertia of the system
is the final moment of inertia of the system
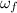 is the final angular velocity
is the final angular velocity
But first, we have to find
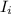 and
and
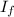 :
:
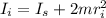 (2)
(2)
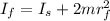 (3)
(3)
Where:
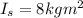 is the student's moment of inertia
is the student's moment of inertia
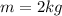 is the mass of each object
is the mass of each object
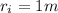 is the initial radius
is the initial radius
 is the final radius
is the final radius
Then:
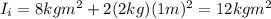 (4)
(4)
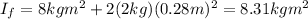 (5)
(5)
Substituting the results of (4) and (5) in (1):
 (6)
(6)
Finding
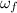 :
:
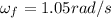 (7) This is the final angular speed
(7) This is the final angular speed
b) Change in kinetic energy:
The rotational kinetic energy is defined as:
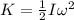 (8)
(8)
And the change in kinetic energy is:
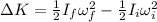 (9)
(9)
Since we already calculated these values, we can solve (9):
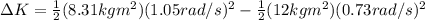 (10)
(10)
Finally:
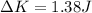 This is the change in kinetic energy
This is the change in kinetic energy