Question

Mr. Jefferson has 20 students in his class. He wants to send 4 of these students to pick up books for the class.

How many combinations of 4 students can he choose?



116,280

80

5

4845

Answer 1

The required "option d) 4845" is correct.

Explanation:

The number of students in the class = 20

Students to pick up books for the class = 4

Here n = 20 and r = 4

To find, the total number of combinations of 4 students can he choose = ?

∴
`^(20)C_4`

`=(20!)/(4!(20-4)!)`

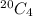
Using the formula,

`^(n)C_r =(n!)/(r!(n-r)!)`

`=(20* 19* 18 * 17 * 16!)/(24* 16!)`

`=(20* 19* 3 * 17)/(4)`

`=5* 19* 51=95* 51`

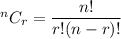
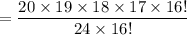
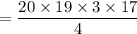
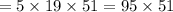
= 4845

The total number of combinations of 4 students can he choose = 4845

Hence, the required "option d) 4845" is correct.