Question

Which pairs of expressions are equal select two. A. 3(x+2) + 4x and 7x+6 B. 2(x +3) + 2x and 4x +3 c. 5x+1) +6 and 5x +5 d 8+4 (x+1) and 4x + 12 e 4+5 (x-2) and 3x+4

Answer 1

Option B, C, E

2(x +3) + 2x and 4x +3 are not equal

(5x+1) +6 and 5x +5 are not equal

4 + 5(x - 2) and 3x + 4 are not equal

Solution:

We have to find the pair of expressions that are equal

Option A

3(x+2) + 4x and 7x+6

Let us simplify the first expression

First expression : 3(x + 2) + 4x

By distributive property, we know that,

a(b + c) = ab + ac

Applying this we get,

3(x + 2) + 4x = 3x + 6 + 4x = 7x + 6

Thus the first and second expressions are equal

Option B

2(x +3) + 2x and 4x +3

Let us simplify the first expression

First expression : 2(x + 3) + 2x

Similarly using distributive property,

2(x + 3) + 2x = 2x + 6 + 2x = 4x + 6

`4x + 6 \\eq 4x + 3`

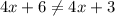
Thus first and second expressions are not equal

Option C

(5x+1) +6 and 5x +5

Let us simplify the first expression

First expression : (5x + 1) + 6

Remove the parenthesis we get,

(5x + 1) + 6 = 5x + 1 + 6 = 5x + 7

`5x + 7\\eq 5x + 5`

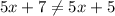
Thus first and second expressions are not equal

Option D

8 + 4(x + 1) and 4x + 12

Let us simplify the first expression

First expression : 8 + 4(x + 1)

Similarly using distributive property,

8 + 4(x + 1) = 8 + 4x + 4 = 4x + 12

Thus first and second expressions are equal

Option E

4 + 5(x - 2) and 3x + 4

Let us simplify the first expression

First expression : 4 + 5(x - 2)

Similarly using distributive property,

4 + 5(x - 2) = 4 + 5x - 10 = 5x - 6

`5x - 6\\eq 3x + 4`

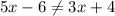
Thus first and second expressions are not equal