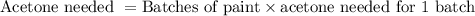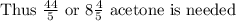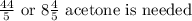
Solution:
Given that,
Lily is making
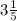 batches of paint
batches of paint
It takes
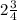 of acetone for one batch
of acetone for one batch
Let us first convert the mixed fraction to improper fraction
Multiply the whole number part by the fraction's denominator
Add that to the numerator
Then write the result on top of the denominator
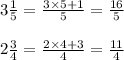
Therefore,
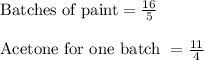
To find the acetone needed for 16/5 batches, multiply it with acetone for one batch