The value of area after 12 years is 1409 square kilometer
Solution:
Given that,
A certain forest covers an area of 2300 square kilometer
Suppose that each year this area decreases by 4%
To find: Area after 12 years
This is decreasing function
The decrease function is given as:
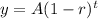
Where,
y is the value after t years
A = initial amount
r = decreasing rate in decimal
t is the number of years
Here in this sum,
A = 2300
t = 12 years
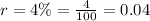
Substituting the values in formula,
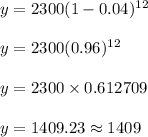
Thus value of area after 12 years is 1409 square kilometer