The first five terms of the sequence is 1, –2, 4, –8, 16.
Solution:
Given explicit formula is
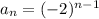 .
.
To find the first five terms of the sequence, substitute n = 1, 2, 3, 4 and 5 in the given explicit formula.
For n = 1, we get
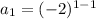
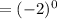
= 1 (Using exponent rule
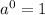 )
)
For n = 2, we get
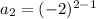
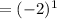

For n = 3, we get
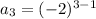
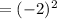
= 4
For n = 4, we get
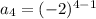
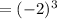
= –8
For n = 5, we get
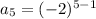
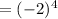
= 16
Hence, the first five terms of the sequence is 1, –2, 4, –8, 16.